


THE PROBLEM of squaring the circle may be described as changing circular into square measure. The mind being trained to estimate in squares, refuses to deal with circles. A plane has a length and breadth, but no thickness. We say a lot is 25 x 100, or a shadow is 10 feet wide by 20 long. We talk about superficial surface without substance. Thickness pertains to the third power and introduces a new dimension which the mind can grasp. A tank 20 x 20 gives no idea of its capacity until we learn that it is 10 feet deep. Then 20 x 20 x 10 shows that it will hold 4,000 cubic feet of water.
If our ancestors had estimated in spheres with the diameter of a small marble as the unit of measurement, we might ail be talking in circles, with the mathematicians trying to circle the square.
For thousands of years they have sought to discover the ratio of the diameter to the circumference as the key to the great problem. Ingenious methods have been employed which produce answers approximately correct, but there, is always an infinitesimally small fraction left over which is technically known as pi.
The ancients claimed the diameter to be one-third of the circumference, and had biblical authority for the same, as we read in the Book of Kings. VII, 23, that Solomon in making the vessels of the Temple “made a molten sea, ten cubits from one rim to the other, and a line of thirty cubits did compass it round about.” This is but one of many references in the Bible to the ratio of the diameter of the circumference being one-third, this ratio gives a fairly good approximation, but for careful estimating we divide the circumference, or multiply the diameter by the decimal fraction 3.141592. From any given diameter we obtain an approximately correct answer to an infinitesimal degree by squaring the radius and multiplying it by pi. It may be said that calculations have been carried out to seven hundred decimal points in the vain hope of hitting upon a cycle of repeating decimals which would close the circuit so as to give a definite value to pi. Here is the process worked out to seven hundred and seven points:
3.1415926535 8979323846 2643383279 5028841971 6939937510
5820974944 5923078164 0628620899 8628034825 3421170679
8214808651 3282306647 0938446095 5058223172 5359408128
4811174502 8410270193 8521105559 6446229489 5493038196
4428810975 6659334461 2847564823 3786783165 2712019091
4564856692 3460348610 4543266482 1339360726 0249141273
7245870066 0631558817 4881520920 9628292540 9171536436
7892590360 0113305305 4882046652 1384146951 9415116094
3305727036 5759591953 0921861173 8193261179 3105118548
0744623799 6274956735 1885752724 8912279381 8301194912
9833673362 4406566430 8602139494 6395224737 1907021798
6094370277 0539217176 2931767523 8467481846 7669405132
0005681271 4526356082 7785771342 7577896091 7363717872
1468440901 2249534301 4654958537 1050792279 6892589235
4201995
This stupendous calculation may be appreciated by explaining that you might imagine a sphere to he constructed with the earth as the central point and the orbit of the circle to extend to the star Sirus, distant a hundred million miles �”but you may make it a billion times farther if you wish�”then imagine this immense sphere packed with minute microbes, so small ‘that a billion could stand on the point of a pin. Now multiply that radius of the great circle by those seven hundred and seven decimals and the error as to the space tilled with microbes will be less than the billionth part of a microbe!
The interest in the squaring of the circle has been kept alive by the offer of 100,000 francs by the Paris Academic of Sciences, and by the claim that the secret was known at the time of the building of the pyramids. The Paris Academie has withdrawn its offer and says it “will examine no more squaring problems, no more trisections of the angle, no more duplications of the cube, and no more perpetual motion schemes.”
The offer was withdrawn because Prof. Gauss gave a rigid and positive demonstration of the impossibility of solving those problems mathematically. Prof. Gauss' proof is too profound and technical for the average student, but for the benefit of the thousand and one aspirants for fame who believe that they have new methods for solving the great problem we will put a new and interesting value to Mr. Shanks’ seven hundred and seven point demonstration as a test wherewith to compare the new solutions. The greatest mathematicians of the world have endorsed his work as correct, and it has been approved by the Royal Society of London, Vol. XXI. All that is necessary to see how far the new methods compare with Mr. Shanks', and at what point they fail: It may he said that out of the hundreds of thousands of mathematicians, many of whom devoted their lives to the task very, very few were correct as far as the fifth or sixth decimal, and here we have an absolutely correct standard up to seven hundred and seven points!
And now for a practical rule and explanation for the benefit of the engineer and mechanic, which when once learned will never be forgotten. To square a circle, multiply half the diameter (in inches) by half the circumference in inches). The answer will be absolutely correct, and not merely approximately near, as many suppose. It is practical, because if you wish to know how many feet of sod will be required to cover a round grass plot, you take the tape, and measure the circumference and the diameter. And multiply the half of one by half the other, and if your measurements are correct it will give the amount of sod required to less than the billionth part of an inch. If the plumber wishes to know the contents of a circular boiler or cistern, let him measure the diameter and the circumference with the tape, then multiply the half of one by half of the other and the result by the depth, and he will have the capacity as close as a single drop of water The correctness of the answer depends altogether on the accuracy of his measurements.
A tinsmith had to cover a circular roof 100 feet in diameter. Actual measurement made the circumference to be 3.1416 feet By the use of this rule he found it required 7,854 feet of tin, which was not a square inch out of the way.
As the reason why has to be taught to impress it upon the mind, we will give a little kindergarten illustration which explains everything. If you halve an orange you will notice how the pulp of the fruit is divided into triangular looking segments of a circle converging to the center B, us shown in the following illustration.
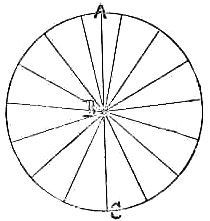
Now let us square that circle of the orange by the rule. Suppose the circumference proves to be 9 3/7 inches, and the diameter 3 inches; the half of the one by the half of the other gives 7 and 1/14 square inches as the correct answer. But why? Suppose the circle was cut in half from A to B, as shown on the circle. Now take each of the pieces of orange and straighten out the peel as shown in the following cut. It is clear that each piece is as long as half of the circumference, because two pieces formed the entire circumference. The pulp is broken or separated into segments which are evidently half as wide the diameter because they reached from every point of the circumference to the center at B. As these triangles taper from the half of the circumference to nil at their points, we will bring the two pieces together so as to fit them into a solid oblong one and a half inches wide by 4 5/7 inches long, containing a surface of 7 and 1/14 inches as stated.
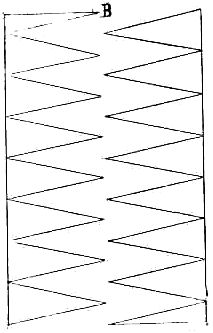
[Page 70]